
380), and Theon's daughter Hypatia (died 415). This system was probably adapted from Babylonian numerals by Hipparchus c. However, the positions were usually limited to the fractional part of a number (called minutes, seconds, thirds, fourths, etc.)-they were not used for the integral part of a number. Hellenistic astronomers extended this into a sexagesimal positional numbering system by limiting each position to a maximum value of 50 + 9 and including a special symbol for zero, which was also used alone like our modern zero, more than as a simple placeholder. A comma or inverted acute is put in front of thousands to distinguish them from the standard use. To represent numbers from 1,000 to 999,999 the same letters are reused to serve as thousands, tens of thousands, and hundreds of thousands. The alphabetic system operates on the additive principle in which the numeric values of the letters are added together to form the total. An acute sign (´) is used to distinguish numerals from letters.

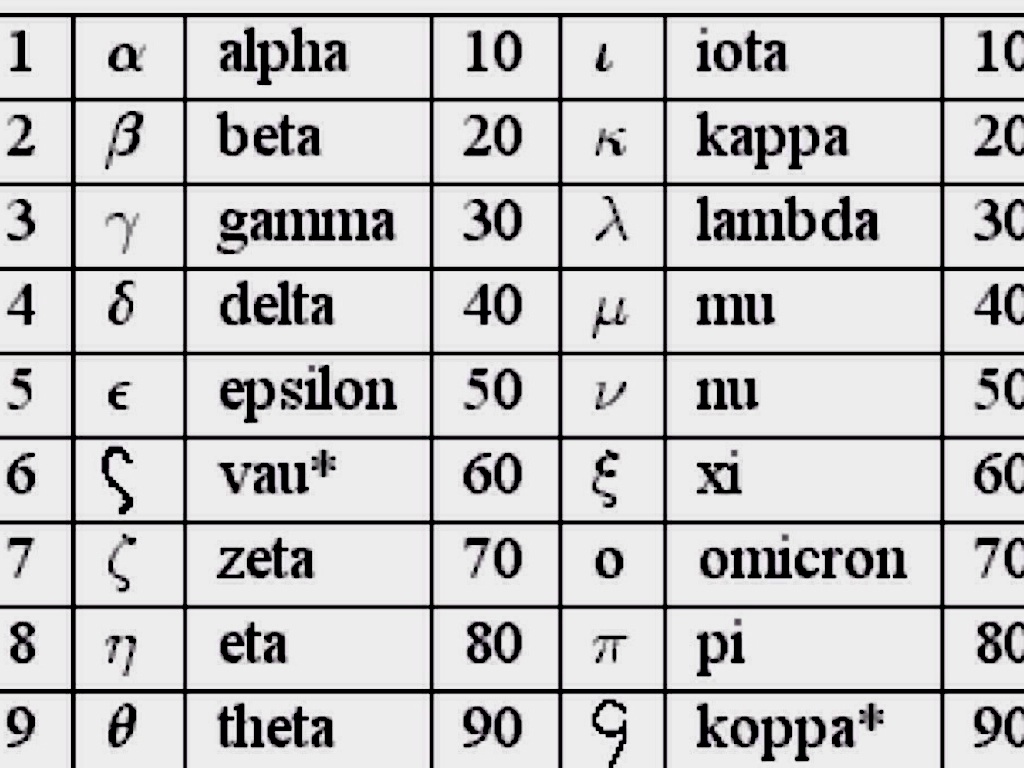
This requires 27 letters, so the 24-letter Greek alphabet was extended by using three obsolete letters: digamma (Ϝ, also used are ς or στ) for 6, qoppa (Ϟ) for 90, and sampi (Ϡ) for 900. , 90) a separate letter, and each hundreds (100, 200. , 9) was assigned a separate letter, each tens (10, 20. Starting in the 4th century BC, the acrophonic system was replaced with a quasi-decimal alphabetic system, sometimes called the Ionic numeral system. The earliest system of numerals in Greek was acrophonic, operating much like Roman numerals (which derived from this scheme), with the following acrophonic formula: Ι = 1, Π = 5, Δ = 10, Η = 100, Χ = 1000, and Μ = 10000. In modern Greece, they are still in use for ordinal numbers, and in much the same situations as Roman numerals are in the West for ordinary numbers, Arabic numerals are used. Template:Table Numeral Systems Greek numerals are a system of representing numbers using letters of the Greek alphabet. ↑ "Systèmes numéraux en Grèce ancienne: description et mise en perspective historique".The Greeks also used the myriad to denote 10,000 (Μʹ) and the myriad myriad for one hundred million (ΜΜʹ). A "left keraia" (Unicode U+0375, ‘Greek Lower Numeral Sign’) is put in front of thousands to distinguish. This alphabetic system operates on the additive principle in which the numeric values of the letters are added together to form the total. To distinguish numerals from letters they are followed by the "keraia" (Greek κεραία- insect antenna), a symbol similar to an acute sign ( Unicode U+0374). This requires 27 letters, so the 24-letter Greek alphabet was extended by using three obsolete letters: fau ϝ, (also used are ϛ or, in modern Greek, ΣΤ) for 6, koppa ϟ for 90, and sampi ϡ for 900. Each unit (1, 2, …, 9) was assigned a separate letter, each tens (10, 20, …, 90) a separate letter, and each hundreds (100, 200, …, 900) a separate letter. The acrophonic system was replaced by a new alphabetic system, sometimes called the Ionic numeral system, from the 4th century BC. The earliest alphabet-related system of numerals used with the Greek letters was a set of the acrophonic Attic numerals, operating much like Roman numerals (which derived from this scheme), with the following formula: Ι = 1, Π = 5, Δ = 10, ΠΔ = 50, Η = 100, ΠΗ = 500, Χ = 1000, ΠΧ = 5000, Μ = 10000 and ΠΜ = 50000. In modern Greece, they are still in use for ordinal numbers, and in much of the same way that Roman numerals are in the West for ordinary ( cardinal) numbers, Arabic numerals are used.Īt first, before it was used more, the Greek alphabet, Linear A and Linear B had used a different system with symbols for 1, 10, 100, 100 operating with the following formula: | = 1, – = 10, ◦ = 100, ¤ = 1000, ☼ = 10000. They are also known by the names Milesian numerals, Alexandrian numerals, or alphabetic numerals. Greek numerals are a system of representing numbers using letters of the Greek alphabet.


 0 kommentar(er)
0 kommentar(er)
